This paper is available on arxiv under CC 4.0 license.
Authors:
(1) Amit Kumar Chatterjee, Yukawa Institute for Theoretical Physics, Kyoto University & Department of Physics, Ramakrishna Mission Vidyamandira;
(2) Satoshi Takada, Department of Mechanical Systems Engineering and Institute of Engineering;
(3) Hisao Hayakawa, Yukawa Institute for Theoretical Physics, Kyoto University.
Table of Links
- Introduction
- Model and Protocol
- Different Regimes in d˜− Γ˜ Plain and Multiple QMPE
- QMPE in Region (d): Second-order Ep
- QMPE in Region (a1): Oscillations
- Summary
- Appendix A: Eigenvectors of L in region (d)
- Appendix B: Eigenvectors of L in region (a1)
- Appendix C: QMPE in region (b): purely exponential relaxation
- Appendix D: QMPE in region (e): 3rd order EP
- References
III. DIFFERENT REGIMES IN d˜− Γ˜ PLANE AND MULTIPLE QMPE
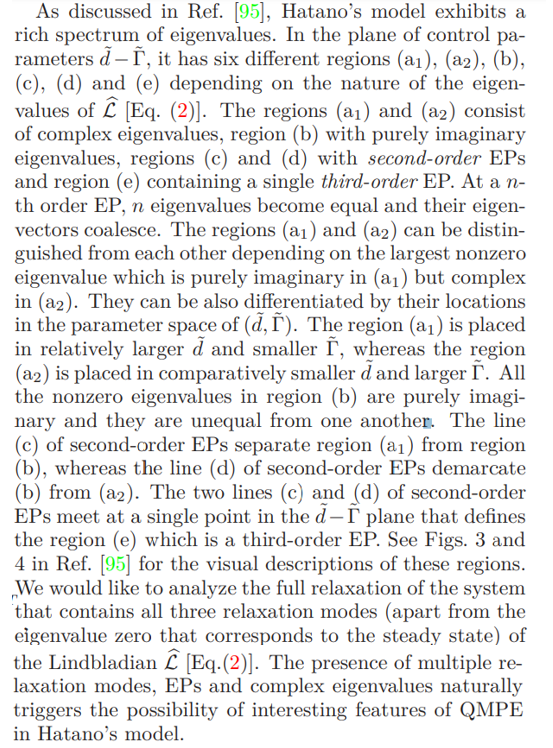
Indeed, in the space of control parameters ( ˜dI, Γ˜ I, ˜dII, Γ˜ II; ˜d, Γ), we find ˜ multiple QMPE where the two trajectories corresponding to some observable, intersect each other multiple times before reaching the same steady state. Specifically, we have obtained two or more intersections in a oscillatory manner, in regions (a1) and (a2) with complex eigenvalues. Whereas, at most two intersections or double QMPE can be found in regions (c), (d) and (e) with EP. Interestingly, even number of intersections (e.g. double QMPE or quadruple QMPE) imply that the initial identities of the two copies (higher valued copy and lower valued copy of an observable, more explicitly hotter and colder copies if the observable is temperature) are restored finally after the last intersection. Therefore, in such cases the initially hotter or higher valued copy remains hotter or higher valued after all the intersections have occurred. On the other hand, odd number of intersections (single QMPE or triple QMPE) indicates the ultimate reversal of the initial identities after the final intersection. In these cases the initially hotter or higher valued copy finally becomes colder or lower valued copy after the completion of all intersections. This classification has important consequences when one is not only interested in faster relaxations or intersections at intermediate times, but also interested in faster reach to final or steady state. It would be intriguing in future to find connections between such odd or even QMPE and faster approach to steady state. Notably, if we restrict on monotonic time volution For that case, even number of QMPE is of no use. Only odd number of QMPE can help to reach faster to steady state using QMPE. However, more conditions are required like avoiding overshoot etc. to use odd QMPE to get faster reach to the steady state. In the next sections, we discuss multiple QMPE in different regimes in detail.
In this paper, we illustrate QMPEs in region (d) for quench onto the second-order EP in Sec. IV (with more analytical details in Appendix A) and region (a1) for the quench onto the point with complex eigenvalues in Sec. V (with more analytical details in Appendix B). We do not discuss QMPE in regions (c) and (a2) separately, because the analyses of QMPEs in these regions are similar to those in regions (d) and (a1), respectively. The QMPEs in other regions are discussed in the appendices. Appendix C discusses QMPE in region (b) with purely exponential relaxations and Appendix D contains the description of the QMPE at the third-order EP in region (e).

