This paper is available on arxiv under CC 4.0 license.
Authors:
(1) Amit Kumar Chatterjee, Yukawa Institute for Theoretical Physics, Kyoto University & Department of Physics, Ramakrishna Mission Vidyamandira;
(2) Satoshi Takada, Department of Mechanical Systems Engineering and Institute of Engineering;
(3) Hisao Hayakawa, Yukawa Institute for Theoretical Physics, Kyoto University.
Table of Links
- Introduction
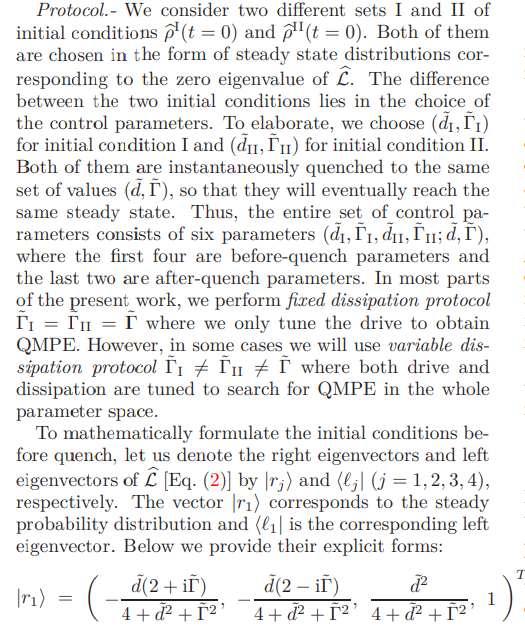
- Model and Protocol
- Different Regimes in d˜− Γ˜ Plain and Multiple QMPE
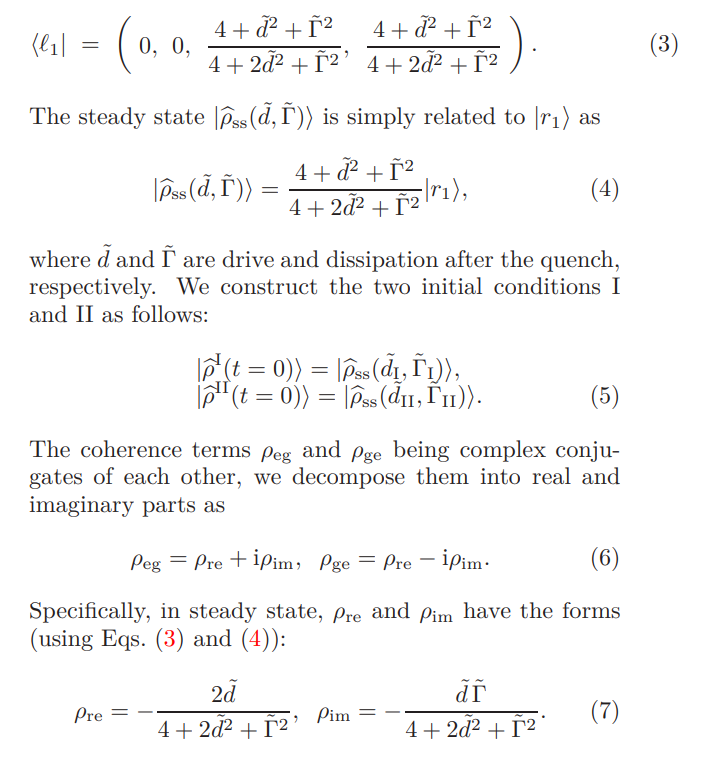
- QMPE in Region (d): Second-order Ep
- QMPE in Region (a1): Oscillations
- Summary
- Appendix A: Eigenvectors of L in region (d)
- Appendix B: Eigenvectors of L in region (a1)
- Appendix C: QMPE in region (b): purely exponential relaxation
- Appendix D: QMPE in region (e): 3rd order EP
- References
II. MODEL AND PROTOCOL
We consider a two-level open quantum system, subjected to a periodic drive and dissipatively coupled to the environment. Following Ref. [95], we hereafter refer to this model as Hatano’s model in the present manuscript. In this paper, we adopt ~ = 1. The two levels of the system, ground state and excited state, have energies Eg and Ee, respectively. The gap between the two levels is denoted by ∆ := Ee − Eg. In Hatano’s model, the transfers between the energy levels are enabled by the external oscillatory electric field E = E0cos(ωt) and the dissipation of the system to the environment via coupling Γ. The parameter δ := ∆ −ω represents the detuning of the periodic drive with respect to the system and d := DE0, where D is the electric dipole moment, denotes the effective electric field. The tuning parameters in Hatano’s model are d, Γ and δ . However, one of them fixes the unit of energy, so we essentially have two free parameters ˜d := d/δ and Γ := Γ ˜ /δ.
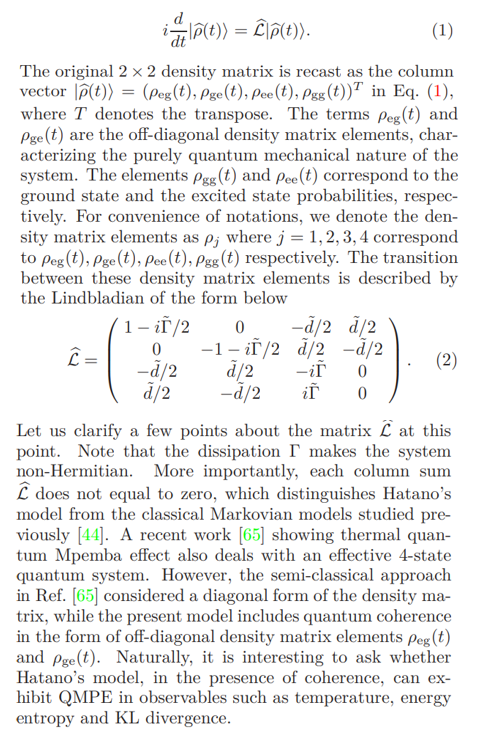
Hatano’s model, within Markovian approximation, can be described by the Gorini-Kossakowski-SudarshanLindblad (GKSL) equation [101–104] (see [95] for the present context):